SIGNIFICANT FIGURE | GENERAL SCIENCE | PHYSICS | ADHYAYAN IAS

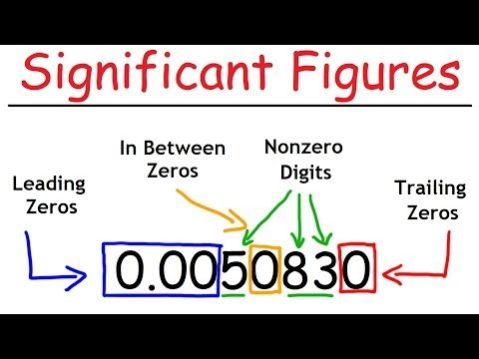
Significant Figures

When a student measures the length of a line as 6.8 cm, the digit 6 is certain, while 8 is uncertain as a little less or more than 0.8 cm is reported as 0.8 cm. Normally those digits in measurement that are known with certainly plus the first uncertain digit, are called significant figures. Thus, there are two significant figures in 1.4 cm. The number of significant figures in any quantity depends upon the accuracy of the measuring instrument. More the number of significant number of figures, less is the percentage of error in the measurement of the quantity. If there are lesser number of significant figures (in a measurement) more will be the percentage error in the measurement. The number of significant figures of a quantity may be found by the following rules:

(i) All non-zero digits are significant. For example, 315.58 has five significant figures.

(ii) All zeros between two non-zero digits are significant. For example,

5300405.003 has ten significant figures.

(iii) All zeros which are to the right of a decimal point and also to the right of a non-zero digit are significant. For example, 50.00 has four significant figures, and so has .04050. It should be noted that in .04050, the first zero to the right of the decimal is not significant but, the last zero is significant.

(iv) All zeros to the right of a decimal point and to the left of a non-zero digit in a decimal fraction are not significant. For example, .00043 has only two significant figures but 2.00023 has 6 significant figures. It is also to be noted that zero conventionally placed to the left of a decimal point is not significant.

(v) All zero to the right of last of non-zero digit are significant, if they come from some measurement. For example, if the distance between two objects is 4050 m (measured to the nearest metre), then 4050 m contains 4 significant figures.

(vi) The number of significant figures does not vary with the change in unit. For example, if the length of an object is 348.6 cm, it has 4 significant figures. If the length is expressed in metre, then it is equal to 3.486 m. Is still has 4 significant figures.

(vii) In a whole number all zeros to the right of the last non zero number are not significant, for example 5000 has only one significant figure.

Importance of significant figures in measurement. As stated earlier, the accuracy of the measurement determines the number of significant figures in the quantity. Suppose the diameter of a coin is 2 cm. If a student measures the diameter with a metre scale which can read up to .1 cm only (i.e. cannot read less than 0.1 cm) the student will report the diameter to be 2.0 cm i.e. upto 2 significant figures only. If the diameter is measured by

an instrument which can read upto .01 cm only (or which cannot measure less than .01cm), viz a Vernier Callipers, he will report the diameter as 2.00 cm i.e. upto 3 significant figure. Similarly if the measurement is made by an instrument like a screw gauge which can measure upto .001 cm only (i.e. cannot measure less than .001 cm), the diameter will be recorded as 2.000 cm i.e. upto 4 significant figures. Thus any measurement should be recorded keeping in view the accuracy of the measuring instrument. Importance of significant figures in expressing the result of calculations Suppose a student measures the side of a cube with the help of a metre scale which comes to be 3.2 cm. He calculates the volume of this cube mathematically and reports it to be (3.2× 3.2× 3.2) cubic centimetre or 32.768 cm3. The reported result is mathematically correct but is not correct in scientific measurement. The correct volume should be recorded as 33 cm3. This is because there are only two significant figures in the length of the side of the cube, hence the volume should also have two significant figures, whereas there are 5 significant figures in 32.768 which is not correct.